JEE 2016 :: Advanced 2016 :: Physics 2016

• Advanced 2016 - Physics 2016
• Advanced 2016 - Chemistry 2016
• Advanced 2016 - Mathematics 2016

Consider an evacuated cylindrical chamber of height h having rigid conducting plates at the ends and an insulating curved surface as shown in the figure. A number of spherical balls made of a light weight and soft material and coated with a  conducting material are placed on the bottom plate. The balls have a  radius r<<h. Now, a high voltage source (HV) connected across the conducting plates such that tjhe bottom plates is at +V₀ and the top plate at -V₀.  Due to their conducting surface, the balls will get a charge, will become equipotential with the plate and are repelled by it. The balls will eventually collide with the top plate, where the coefficient of restitution can be taken to be zero due to the soft nature of the material of the balls. The electric field in the chamber can be considered to be that of a parallel plate capacitor. Assume that there are no collisions between the balls and interaction between them is negligible.(Ignore gravity)

The average current in the steady state registered by the ammeter in the circuit will be

Answer:

Explanation:

As the balls keep on carrying charge form one plate to another, current will keep on flowing even in steady state. When at bottom plate, if all balls attain charge q,

$\frac{kq}{r}=V_{0}$

                                  $(k= \frac{1}{4\pi\epsilon_{0}})$

$\Rightarrow        q=\frac{V_{0}r}{k}$

Inside cyclinder, electric field,

$E= [ V_{0}-(-V_{0})]h=2V_{0}h.$

$\Rightarrow$ Acceleration of each ball,

$a=\frac{qE}{m}=\frac{2hr}{km}.V_{0}^{2}$

$\Rightarrow$  Time taken by balls to each other plate,

$t=\sqrt{\frac{2h}{a}}=\sqrt{\frac{2h.km}{2hrV_0^2}}=\frac{1}{V_{0}}\sqrt{\frac{km}{r}}$

 If there are n balls, 

  then Average current,

$i_{av}=\frac{nq}{t}=n\times \frac{V_{0}r}{k}\times V_{0}\sqrt{\frac{r}{km}}$

$\Rightarrow  i_{av}\propto V_{0}^{2}$

A frame of the reference that is accelerated with respect to an inertial frame of reference is called a non- inertial frame of reference. A coordinate system fixed on a circular disc rotating about a fixed axis with a constant angular velocity ω is an example of a non-inertial frame of reference. The relationship  between the force  $\overrightarrow{F}_{rot}$  experienced by a particle of mass m moving on the  rotating disc and the force  $\overrightarrow{F}_{in}$ experienced by the particle in an inertial frame of reference is,

$\overrightarrow{F}_{rot}= \overrightarrow{F_{in}}+2m(\overrightarrow{v}_{rot}\times \overrightarrow{ \omega})+ m(\overrightarrow{\omega}\times \overrightarrow{r})\times\overrightarrow{\omega}$

  where, V_rot is the velocity of the particle in the rotating  frame of reference and r is the position vector of the particle with respect to center of the disc

Now, consider a smooth slot along a diameter of a disc of radius R  rotating counter-clockwise with a constant angular speed ω about its vertical axis through its centre. We assign a coordinate system with the origin at the centre of the disc, the X-axis along the slot, the Y-axis perpendicular to the slot and Z-axis along the rotation axis($(\omega =\omega\hat{k})$. A small block of mass m is gently placed in the slot at $r=(\frac{R}{2})\hat{i}$ at t=0 and is constrained to move only along the slot

The net reaction  of the disc  on the block is

Answer:

Explanation:

$F_{rot}=F_{in}+2m(v_{rot}\hat{i})\times \omega \hat{k}+m(\omega \hat{k}\times r\hat{i})\times \omega \hat{k}$

$mr\omega^{2}\hat{i}=F_{in}+2 mv_{rot}\omega (-\hat{j)}+m\omega^{2}r\hat{j}$

$F_{in}=2mv_{r}\omega \hat{j}$

$r=\frac{R}{4}[e^{\omega t}+e^{-\omega t}]$

 $\frac{\text{d}r}{\text{d}t}=v_{r}=\frac{R}{4}[\omega e^{\omega t}- \omega e^{-\omega t}]$

$F_{in}=2m\frac{R\omega}{4}[e^{\omega t}-e^{-\omega t}]w\hat{j}$

 $F_{in}=\frac{mR\omega^{2}}{2}[e^{\omega t}-e^{-\omega t}]\hat{j}$

Also , reaction  is due to disc surface , then

 $F_{reaction}=\frac{mR\omega^{2}}{2}[e^{\omega t}-e^{-\omega t}]\hat{j}+mg\hat{k}$

A frame of the reference that is accelerated with respect to an inertial frame of reference is called a non- inertial frame of reference. A coordinate system fixed on a circular disc rotating about a fixed axis with a constant angular velocity ω is an example of a non-inertial frame of reference. The relationship between the force $\overrightarrow{F}_{rot}$ experienced by a particle of mass m moving on the rotating disc and the force $\overrightarrow{F}_{in}$ experienced by the particle in an inertial frame of reference is,

$\overrightarrow{F}_{rot}= \overrightarrow{F_{in}}+2m(\overrightarrow{v}_{rot}\times\overrightarrow{\omega})+ m(\overrightarrow{\omega}\times \overrightarrow{r})\times\overrightarrow{\omega}$

where $V_{rot}$ is the velocity of the particle in the rotating frame of reference and r is the position vector of the particle with respect to center of the disc

Now, consider a smooth slot along a diameter of a disc of radius R  rotating counter-clockwise with a constant angular speed ω about its vertical axis through its centre. We assign a coordinate system with the origin at the centre of the disc, the X-axis along the slot, the Y-axis perpendicular to the slot and Z-axis along the rotation axis $(\omega =\omega\hat{k})$. A small block of mass m is gently placed in the slot at $r=(\frac{R}{2})\hat{i}$ at t=0 and is constrained to move only along the slot

The distance r of the block  at time t is

Answer:

Explanation:

Force on block along slot=$m\omega^{2}r$

  $=ma=m(\frac{vdv}{dr})$

$\int_{0}^{v}vdv =\int_{R/2}^{r}\omega^{2} rdr$

$\Rightarrow\frac{v^{2}}{2}=\frac{\omega^{2}}{2}(r^{2}-\frac{R^{2}}{4})$

$v=\omega \sqrt{r^{2}-\frac{R^{2}}{4}}=\frac{\text{d}r}{\text{d}t}$

$\Rightarrow \int_{R/4}^{r} \frac{\text{d}r}{\sqrt{r^{2}-\frac{R^{2}}{4}}}=\int_{0}^{t}\omega dt $

$ln[\frac{r+\sqrt{r^{2}-\frac{R^{2}}{4}}}{\frac{R}{2}}$

- $ln[\frac{\frac{R}{2}and+\sqrt{\frac{R^{2}}{4}-\frac{R^{2}}{4}}}{\frac{R}{2}}]=\omega t$

$\Rightarrow r+\sqrt{r^{2}-\frac{R^{2}}{4}}=\frac{R}{2}e^{\omega t}$

$\Rightarrow r^{2}-\frac{R^{2}}{4}=\frac{R^{2}}{4}e^{2\omega t}+r^{2}-2r\frac{R}{2}e^{\omega t}$

$\Rightarrow r=\frac{\frac{R^{2}}{4}e^{2\omega t}+\frac{R^{2}}{4}}{Re^{\omega t}}=\frac{R}{4}(e^{\omega t}+e^{-\omega t})$

Light  of wavelength $\lambda_{ph}$ falls on a  cathode plate inside a vacuum tube as shown in the figure. The work function of the cathode surface is Φ and the anode is a wire mesh of conducting material kept at a distance d from the cathode. A potential difference V is maintained between the electrodes. If the minimum de-Broglie wavelength of the electrons passing through  the anode is $\lambda_{e}$ . which of the following statement (s) is (are) true ?

Answer:

Explanation:

$k_{max}=\frac{hc}{\lambda_{ph}}-\phi$

Kinetic energy of electron reaching the anode will be

$K=\frac{h_{c}}{\lambda_{ph}}-\phi+eV$

Now $\lambda_{e}=\frac{h}{\sqrt{2mK}}$

  $= \frac{h}{\sqrt{2m(\frac{hC}{\lambda_{ph}}-\phi+eV)}}$

If eV>>Φ

     $\lambda_{e}= \frac{h}{\sqrt{2m(\frac{hC}{\lambda_{ph}}+eV)}}$

If  $V_{f}=4V_{i}, (\lambda_{e)}f\approx (\lambda_{e)i}$

In the circuit shown below, the key is  pressed at time t=0, Which of the following statement(s) is (are)  true?

Answer:

Explanation:

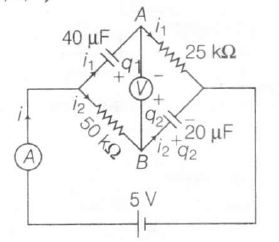
Just after pressing key,

  5-25000i₁ =0

5-50000 i₂ =0

 ( As charge in both capacitors =0)

$\Rightarrow i_{1}=0.2mA\Rightarrow i_{2}=0.1mA$

And  $V_{B}+25000i_{1}=V_{A}$

$\Rightarrow V_{B}-V_{A}=-5V$

After a long time , i₁  and i₂ =0 ( steady state)

$\Rightarrow 5-\frac{q_{1}}{40}=0\Rightarrow q_{1}=200\mu C$

and  $ 5-\frac{q_{2}}{20}=0\Rightarrow q_{2}=100\mu C$

$V_{B}-\frac{q_{2}}{20}=V_{A}\Rightarrow V_{B}-V_{A}=+5 V$

$\Rightarrow$ (a) is correct.

For capacitor 1,

$q_{1}=200[1-e^{-\frac{t}{l}}]\mu C\Rightarrow i_{1}=\frac{1}{5}e^{-\frac{t}{l}}mA$

For capacitor 2,

$q_{2}=100[1-e^{-\frac{t}{l}}]\mu C\Rightarrow i_{2}=\frac{1}{10}e^{-\frac{t}{l}}mA$

$\Rightarrow V_{B}-\frac{q_{2}}{20}+i_{1}\times 25=V_{A}$

$\Rightarrow V_{B}-V_{A}=5[1-e^{-t}]-5e^{-t}$

     $=-5[1-2e^{-t}]$

 At t=ln 2,

  $V_{B}-V=5[1-1]=0$

$\Rightarrow$ (b) is correct.

At t=1,

 $i=i_{1}+i_{2}=\frac{1}{5}e^{-1}+\frac{1}{10}e^{-1}=\frac{3}{10}.\frac{1}{e}$

At t=0

$i=i_{1}+i_{2}=\frac{1}{5}+\frac{1}{10}=\frac{3}{10}$

$\Rightarrow$ (c) is correct

 After a long time , $i_{1}=i_{2}=0$

$\Rightarrow$ (d) is correct.

• Advanced 2016 - Physics 2016
• Advanced 2016 - Chemistry 2016
• Advanced 2016 - Mathematics 2016